Sign in Subscribe
Physics

Our Curling Earth And Its Magnetic Field

Andres

• 6 min read
The Earth rotating in its orbit and geomagnetism
Photo by NASA / Unsplash

In our solar system, it is a well known fact that Earth is the third closest planet to the sun, dragged around it in the distinct eliptical orbit that gives us different seasons. To the every-day viewer, its yearly trawl around the sun is a slow one, steadily travelling around it until it closes the loop 365 days after starting it. To the average physicist, however, it almost feels like we should be celebrating that it is stationary relative to us - for we would be sent flying at over 100,000 km/h (the Earth's orbiting speed) if it weren't. I think it would be exhilarating, though!

In any case, to the average scientist, the earth is also rather spherical. Not perfectly spherical, mind you, but enough that approximating its shape to a colossal ball handily allows us to explain a variety of phenomena taking place all around us. In our case, we will look at the geomagnetic field. Most people, I believe, will already know a couple factoids about it, like how it influences direction compasses or how it plays a role in monarch butterfly migrations and magnetoreception (shameless self-promotion here), but not many will actually know about what it is.

Directional compass and navigations
Photo by Denise Jans / Unsplash

Just a quick disclaimer before I start. I understand that this topic can get very mathematical very quickly, but in the interest of simplicity and efficiency, I will not be including anything of the sort here (though I may refer to it). Let's begin, then.

Planets Are Like Onions

It is true. Earth, or at least what diagrams and artistic representations make of it, bears a striking resemblance to an onion - namely, both have layers. What distinguishes them, of course, is that we give names and categories to our planet's layers. The topmost layer is known as the lithosphere, and it is made up of the earth's crust (where we stand on) and its upper mantel, harbouring the tectonic plates that produce earthquakes as they move. Deeper within the mantel is the silicate-based athenosphere, below which magma starts forming, and around 2900 km deep into the earth we get the outer core. Now, here is where things get interesting.

Overall, the core of Earth is just about the size of Mars, and it is constituted of two parts: the inner core and the outer core. The outer core is a great turmoil of flowing convection currents mostly composed of molten iron, nickel and sulfur, with a couple other elements distributed in between. Temperatures here can get as low as 3,530 °C (or 6,380 °F), with pressures that are calculated to be over a thousand times atmospheric pressure (enough that you would be squished into a pancake very quickly). The inner core gets even weirder. At about 5,100 km into the earth, this place is almost entirely made up of pure, solid iron. This essentially means that even its enormous temperatures of 5,730 °C (10,340 °F) are not enough to melt the iron due to the astronomical pressure compacting it together.

Remarkably, it would appear that the inner core is also expanding over time, as molten iron from the outer core is freezing and solidifying to add onto it. This might seem counter-intuitive (especially since the temperatures we're referring to aren't normall associated with freezing objects), but it is simply due to the heat constantly being released into space as radiation by the earth. This cools the planet down enough that the pressure in the outer core can supersede the temperature, thereby making the liquid inside turn solid. Think about my previous point, though: the outer core is made up of molten iron, nickel and sulfur. So, what happens to the materials beside the iron? Basically, as the iron freezes and leaves the mixture at the inner core boundary, the resulting fluid is left with less heavy material and hence becomes more buoyant. In turn, this makes the materials around the boundary rise perpendicular to it, with different plumes of fluid joining together to result in what might be likened to a long, rotating pillar parallel to the north-south polar axis (an effect caused by the conservation of angular momentum). You could imagine it as corkscrew spikes of magma shooting out from the iron ball that is the inner core. This process is called compositional convection, and the rotation of the Earth further contributes to their rotation.

So, now that we have outer core convection currents and curling fluid pillars in mind, we move on to the main topic of this post.

The Geomagnetic Field

As we have seen so far, the earth's core contains a fairly chaotic mess of loops and spinning plumes. Through research and with modern technology, researchers have found even more loops and curls in the core, though of a different sort. And, just like me, it amazes them.

As rule, whenever an electric current is flowing, a magnetic field proportional to it is created on a plane perpendicular to the direction of the current (Ampére's circuital law). Since this implies that the geomagnetic field was first produced by giant electric currents, scientists did their usual thing and promulgated a variety of different theories about how it came to be. Personally, I think the electricity was probably just a product of the chaotic nature of our planet in its early stages, what with all the chemical reactions and thermal changes occuring in its mantel at the time. Either way, a magnetic field was formed. A property of magnetic fields is that, depending on the conductivity of a fluid, they can be 'dragged' along by the fluid as it moves in space (Alfvén's theorem). This field of study is known as magnetohydrodynamics, and here is the part where I say "just trust me." Though I do recommend looking the matter up sometime, do be warned that it assumes a very hefty chunk of experience in multivariable calculus and other topics in uni-grade mathematics - not something I really want to focus on here.

Anyway. In the outer core, the aforementioned convection currents 'drag' the magnetic field lines parallel to the polar axis, travelling so quickly that, together, they form elipses of magnetic fields that sandwich the inner core on top and below it. The weaker rotating pillars created by compositional convection at the inner core boundary then rise up to meet these magnetic field loops and, similarly, they 'drag' the field lines and transfer their rotation to them. This results in the magnetic field sandwich obtaining smaller loops within itself (loop-ception), and here is where I give you another law of electrodynamics.

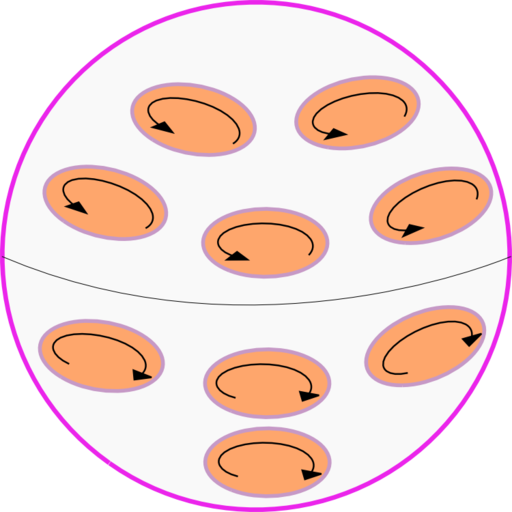
Dynamo theory and how the Earth's magnetic field is generated
Image taken from Wikipedia Commons

Faraday's law states that all magnetic fields can generate an electric field (and hence a current) that is proportional and perpendicular to them. This is exactly what happens with the core's magnetic fields - the small field loops generate electric currrents that add up one-by-one, until the giant encompassing field elipses have currents that look practically identical to the original sandwich shape described. When Ampére's circuital law is applied again, it can be shown that these currents produce further Earth-size magnetic fields - and these are the typical geomagnetic fields that we picture when speaking of them. Phew!

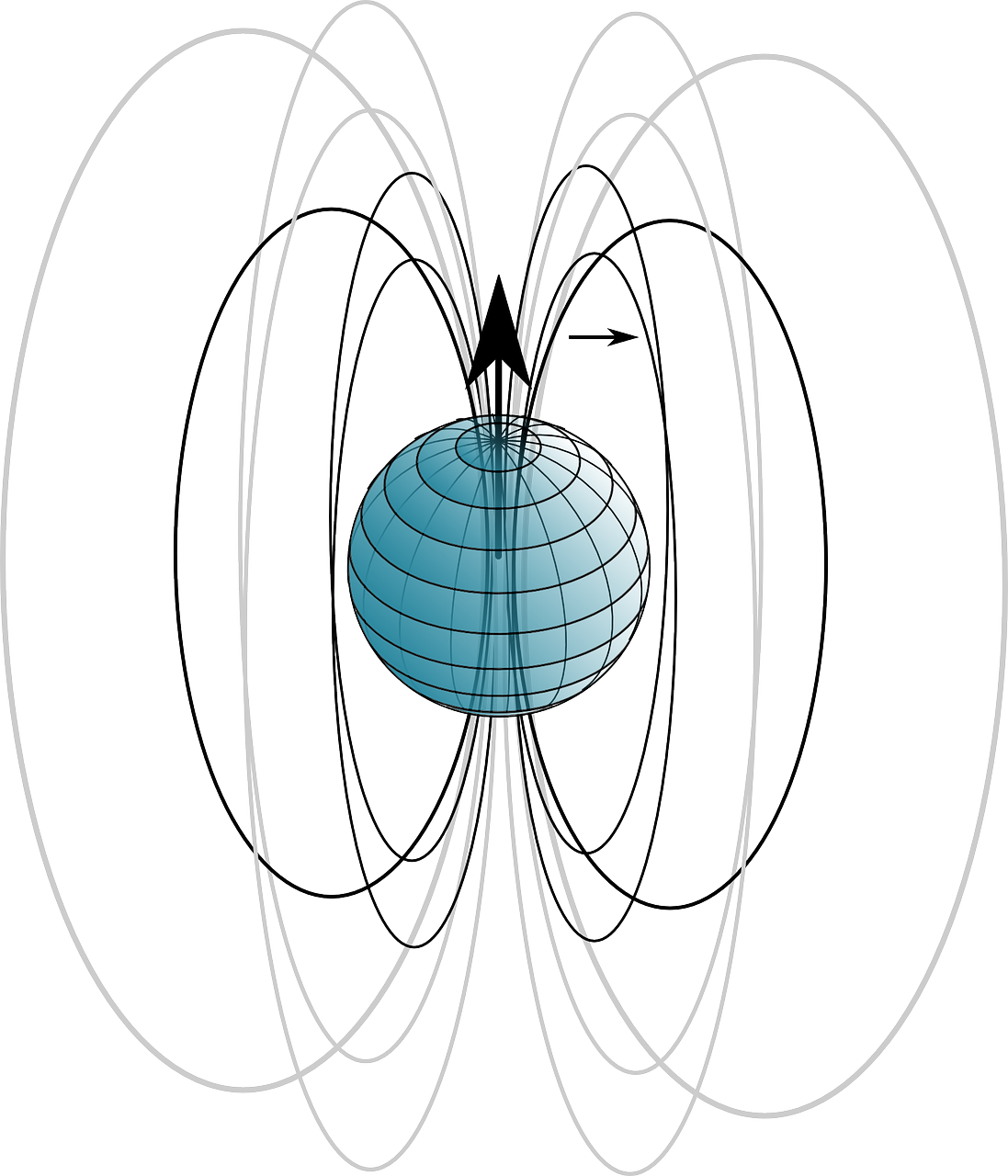
The geomagnetic field through the north-south polar axis
Image taken from Pixabay

(As a sidenote, this back-and-forth generation of magnetic and electric fields is not an endless cycle that repeatedly adds onto itself, as this would be creating energy from nothing and would contradict everything we know about the universe. Instead, as Lentz's law states, electric fields oppose the magnetic fields that create them, which eventually negates the fields completely if they are not renewed.)

First proposed by German physicist Walter Maurice Elsasser in 1946, the fluid-electrodynamic pathways talked about here ultimately led to the theory of the geomagnetic field's generation being called the dynamo theory. Though this is only a small part of the universe, observing our home planet makes for amazing opportunites to more accurately understand the general laws governing our reality - making it easier to predict the even greater magnetic fields produced by terrestial objects like our sun and other stars in our galaxy. What's more amazing is how smoothly all of those laws integrate with each other; enough to be humbled by it, I would say.

References

Sign up for more like this.

Enter your email
Subscribe